【算法笔记】贪心

贪心算法

贪心与其说是一种算法，不如说一种思想。

贪心思想，顾名思义，就是总是做出当前最好的选择，这种方式可能在全局上不是最好的结果，但是在一些题目中就可以直接用。

最简单的例子就是“货比三家”，在生活中，我们买东西时都会挑性价比最优的，这就是一种贪心。

贪心算法在OI中经常与其他算法或数据结构结合到一起，有些题目比较考验思维能力。

贪心算法一般会用到的算法或数据结构不多，一般的题目只需要用到排序，有些题目可以用优先队列动态维护最值。

接下来我们介绍几种贪心算法的“套路”。

取最优

最经典的问题就是最优装载问题。

商店里有$n$个物品，每个物品给出价值和重量，现在来了个小偷，小偷只能带走$m$千克的物品，问他最多能带走多少钱的物品。

对于这道题，我们可以直接算出每一个物品的性价比，然后对于性价比排序，依次累加，直到重量超过$m$为止。

代码

struct node{
	int w,m;//价值和重量
	double d;//性价比
}a[N];
bool cmp(node a,node b){
	return a.d>b.d;//按照性价比从小到大排序
}
int n,m;
int main(){
	cin>>n>>m;//n个物品，限定m重量
	for(int i=1;i<=n;i++) cin>>a[i].w>>a[i].m,a[i].d=(double)a[i].w/(double)a[i].m;
	sort(a+1,a+1+n,cmp);//排序
	int s1=0,s2=0;//分别为价值的累计和重量的累计
	for(int i=1;i<=n;i++){
		s1+=a[i].w,s2+=a[i].m;
		if(s2+a[i+1].m>m) break;//如果超出m限制，就退出
	}
	cout<<s1;//输出累计价格
}

不相交区间问题

在一个数轴上有n条线段，现要选取其中k条线段使得这k条线段两两没有重合部分,问最大的k为多少?

关于这题的贪心策略，我们可以按每个区间的右端点排序，然后每个区间判断一下是否与前面的区间重合，累加答案即可。

代码

struct node{
	int l,r;//结构体
};
vector<node>v; 
int n,ans=0;
bool cmp(node a,node b){
	return a.r<b.r;//按右端点排序
}
int main(){
	cin>>n;
	while(n--){
		int l,r;
		cin>>l>>r;
		v.push_back({l,r});//使用vector容器
	}
	sort(v.begin(),v.end(),cmp);
	int l=v.front().l;//初始l为第一个区间的左端点
	for(auto it:v){
		if(it.l>=l){//如果不重合，就累加答案
			ans++;
			l=it.r;//更新l指针
		}
	}
	cout<<ans;
}

区间选点问题

给定$N$个区间$[a,b]$，取尽量少的点，使得每个区间内都至少有一个点（不同区间内含的点可以重复）。

如何能选取更少的点呢？

如果在几个区间的重叠部分放点，那么就会覆盖多个区间，就会少取一些点。

如图，如果两个区间有重叠部分，那么前面区间的右端点肯定在重叠部分里。

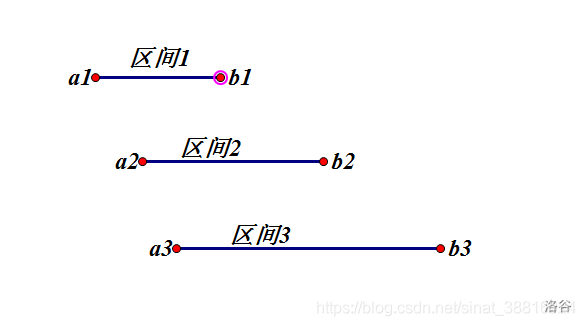
所以我们可以选择在每个区间的右端点放点，这样就会覆盖更少的区间。

所以我们的贪心策略就是：首先按区间的结束位置从小到大排序。然后从区间$1$到区间$n$进行选择；对于当前区间，若集合中的数不能覆盖它，则将区间末尾的数加入集合。

代码

int vis[N];
struct node{
	int u,v,w;
}a[N];
int m,h,ans=0;
int cmp(node a,node b){
	return a.v<b.v;//按照右端点排序
}
int main(){
	cin>>m>>h;
	for(int i=1;i<=h;i++) cin>>a[i].u>>a[i].v>>a[i].w;
	sort(a+1,a+1+h,cmp);//排序
	for(int i=1;i<=h;i++){
		int cnt=0;
		for(int j=a[i].u;j<=a[i].v;j++) cnt+=vis[j];
		if(cnt>=a[i].w) continue;
		for(int j=a[i].v;j>=a[i].u,cnt<a[i].w;j--){
			if(!vis[j]) cnt++,vis[j]++,ans++;
		}
	}	
	cout<<ans;
}

中位数

在贪心算法中，中位数是一个比较神奇的性质。

我们以经典的货仓选址问题为例。

在一条数轴上有 $N$ 家商店，它们的坐标分别为 $A_1∼A_N$。现在需要在数轴上建立一家货仓，每天清晨，从货仓到每家商店都要运送一车商品。求把货仓建在何处，可以使得货仓到每家商店的距离之和最小。

可以证明，当选取中位数时，距离之和最小。

代码

int a[N],sum,n;
int main(){
    cin>>n;
    for(int i=1;i<=n;i++) cin>>a[i];
    sort(a+1,a+n+1);
    int mid=a[n/2];
    for(int i=1;i<=n;i++) sum+=abs(a[i]-mid);
    cout<<sum<<endl;
}

与优先队列相结合

很多贪心题目可以和堆结合到一起，在平时做题时，可以使用stl中的优先队列priority_queue。

合并果子是一道比较经典的优先队列优化贪心题。

有$n$个果子，每次可以任意选择两个进行合并，每次合并耗费的体力值是两个果子重量之和，问最小耗费体力值。

因为是任意两个进行合并，所以我们可以每次选择果子里面重量最小的两个果子合并。

但是暴力找重量最小的果子复杂度为$O(n)$，总体$O(n^2)$的时间复杂度不能接受。考虑用数据结构优化。

什么数据结构能支持动态找最小值呢？堆！

在这里我们可以使用stl中的优先队列，这样就有了$O(n\log n)$的复杂度。

代码

#include<bits/stdc++.h>
using namespace std;
priority_queue<int,vector<int>,greater<int>>q;//优先队列
int n,ans=0;
int main(){
	cin>>n;
	for(int i=1;i<=n;i++){
		int t;
		cin>>t;
		q.push(t);//在优先队列中加入所有果子
	}
	while(!q.empty()){
		int t1=q.top();//取出当前重量最小的果子
		q.pop();
		if(q.empty()) break;
		int t2=q.top();
		q.pop();
		ans+=t1+t2,q.push(t1+t2);
	}
	cout<<ans;
}

反悔贪心

没人看的，所以咕了

例题

一本通提高篇的几道贪心水题。

【一本通提高篇贪心】 活动安排

题目链接

题目大意

选取最多的区间，使它们没有重叠部分。

贪心思路

按照活动的结束时间进行升序排序，因为一个活动结束的越早，就越不容易与其他活动起冲突。

这题就是上面写到的不相交区间问题，这里就不仔细讲了，直接看代码。

AC代码

#include<bits/stdc++.h>
using namespace std;
const int N=1010;
struct node{
	int l,r;//结构体
};
vector<node>v; 
int n,ans=0;
bool cmp(node a,node b){
	return a.r<b.r;//按右端点排序
}
int main(){
	cin>>n;
	while(n--){
		int l,r;
		cin>>l>>r;
		v.push_back({l,r});//使用vector容器
	}
	sort(v.begin(),v.end(),cmp);
	int l=v.front().l;//初始l为第一个区间的左端点
	for(auto it:v){
		if(it.l>=l){//如果不重合，就累加答案
			ans++;
			l=it.r;//更新l指针
		}
	}
	cout<<ans;
}

【一本通提高篇贪心】 种树

题目链接

题目大意

即刚刚讲过的区间选点问题。

贪心思路

按每个路段的右端点排序，枚举每一个路段，从左端点枚举到右端点。

因为如果右端点重叠了，就少种了一棵树，所以只要这个位置有树，这个路段要求的树的数量就-1。

AC代码

#include <bits/stdc++.h>
using namespace std;
const int N=3e4+10;
int vis[N];
struct node{
	int u,v,w;
}a[N];
int m,h,ans=0;
int cmp(node a,node b){
	return a.v<b.v;
}
int main(){
	cin>>m>>h;
	for(int i=1;i<=h;i++) cin>>a[i].u>>a[i].v>>a[i].w;
	sort(a+1,a+1+h,cmp);
	for(int i=1;i<=h;i++){
		int cnt=0;
		for(int j=a[i].u;j<=a[i].v;j++) cnt+=vis[j];
		if(cnt>=a[i].w) continue;
		for(int j=a[i].v;j>=a[i].u,cnt<a[i].w;j--){
			if(!vis[j]) cnt++,vis[j]++,ans++;
		}
	}	
	cout<<ans;
}

【一本通提高篇贪心】 喷水装置

题目链接

题目大意

有 $n$ 个浇灌喷头。每个喷头都装在草坪中心线上（离两边各$\frac{W}{2}$米）。我们知道每个喷头的位置（离草坪中心线左端的距离），以及它能覆盖到的浇灌范围。

请问：如果要同时浇灌整块草坪，最少需要打开多少个喷头？

贪心思路

这题怎么这么计几啊？

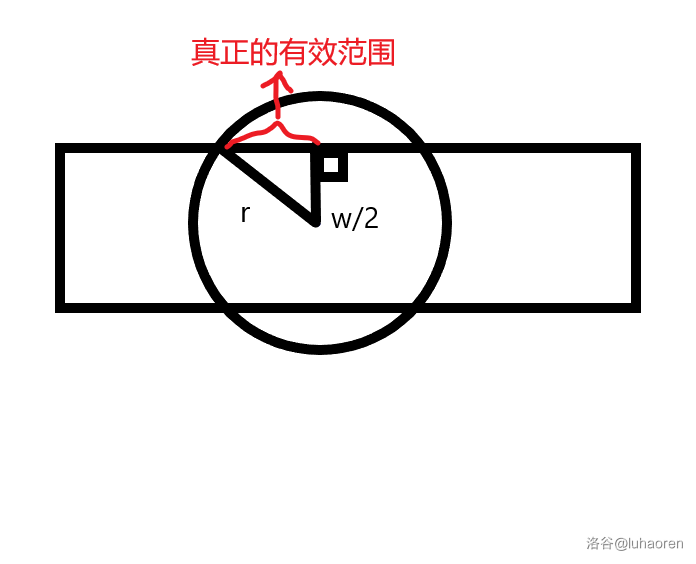
如图，每个喷水装置覆盖的区域不是它的直径，我们需要用勾股定理算出那个圆和那个方块的交集的距离，即$\sqrt{r^2-(\frac{w}{2})^2}$。

算出这个距离后，就是一道裸的区间覆盖问题了。

所以我们的贪心思路就是，先处理处每一个区间的左右端点（即$x-\sqrt{r^2-(\frac{w}{2})^2}$和$x+\sqrt{r^2-(\frac{w}{2})^2}$），然后按左端点排序，依次处理即可，细节部分看代码。

AC代码

#include <bits/stdc++.h>
using namespace std;
const int N=15010;
int T,n,m;
double l,w;
struct node{
	double l,r;
}a[N];
bool cmp(node a,node b){
	return a.l<b.l;
}
void work(){
	m=0;
	cin>>n>>l>>w;
	for(int i=1;i<=n;i++){
		double id,r;
		cin>>id>>r;
		if(r<=w/2) continue;
		double d=sqrt(r*r-w*w/4.0);
		a[++m]={id-d,id+d};
	}
	sort(a+1,a+1+m,cmp);
	double now=0;
	int cnt=0,i=1;
	while(now<l){
		cnt++;
		double tmp=now;
		while(a[i].l<=tmp&&i<=m) now=max(now,a[i].r),i++;
		if(tmp==now&&tmp<l){
			cout<<-1<<endl;
			return;
		}
	}
	cout<<cnt<<endl;
}

int main(){
	cin>>T;
	while(T--) work();
	return 0;
}

【一本通提高篇贪心】 加工生产调度

题目链接

题目大意

有$n$个物品需要加工，每个物品先在$A$车间加工再到$B$加工，问最短加工时间。

贪心策略

按每个物品在$A$和$B$车间的加工时间的最小值排序，然后模拟即可。

AC代码

#include <bits/stdc++.h>
using namespace std;
const int N=1010;
int n;
struct node{
	int a,b,id;
}a[N];
int w[N];
bool cmp2(node a,node b){
	return min(a.a,b.b)<min(a.b,b.a);
}
bool cmp1(node a,node b){
	return min(a.a,a.b)<min(b.a,b.b);
}
int main(){
	cin>>n;
	for(int i=1;i<=n;i++) cin>>a[i].a,a[i].id=i;
	for(int i=1;i<=n;i++) cin>>a[i].b;
	sort(a+1,a+1+n,cmp1);
	for(int i=1,l=0,r=n+1;i<=n;i++){
		if(a[i].a<a[i].b) w[++l]=a[i].id;
		else w[--r]=a[i].id;
	}
	int s1=0,s2=0;
	sort(a+1,a+1+n,cmp2);
	for(int i=1;i<=n;i++){
		s1+=a[i].a;
		s2=max(s1,s2);
		s2+=a[i].b;
	}
	cout<<s2<<endl;
	for(int i=1;i<=n;i++) cout<<w[i]<<" ";
}

【一本通提高篇贪心】 智力大冲浪

题目链接

题目大意

有一些任务，每个任务都有完成期限，完不成一个任务就要扣一些钱，问最多能得到多少钱。

贪心策略

直觉上，如果要扣钱最少，肯定得让扣钱多的排到前面去，然后按照时间依次完成任务。

但是这样做是错误的（通过样例就能得出结论），所以我们需要优化一下这个思路。

我们可以用最优贪心，每个任务都在完成期限的最后一秒完成，用一个数组记录下每个时间点有没有被一个任务占用，然后向前找到最后一个能用的时间即可。

AC代码

#include <bits/stdc++.h>
using namespace std;
const int N=510;
struct node{
	int t,x;
}a[N];
bool cmp(node a,node b){
	return a.x>b.x;
}
int n,m,tim[N];
int main(){
	cin>>m>>n;
	for(int i=1;i<=n;i++) cin>>a[i].t;
	for(int i=1;i<=n;i++) cin>>a[i].x;
	sort(a+1,a+1+n,cmp);
	for(int i=1;i<=n;i++){
		for(int j=a[i].t;j;j--){
			if(!tim[j]){
				tim[j]=1,a[i].x=0;
				break;
			}
		}
	}
	for(int i=1;i<=n;i++) m-=a[i].x;
	cout<<m;
}

【一本通提高篇贪心】 数列极差

题目链接

题目大意

有$n$个正整数，每次删去两个数$a,b$，放入一个数$a\times b+1$，问最后得到的一个数的最大值和最小值。

贪心策略

我们会发现这题很像之前的例题果子合并，所以我们可以考虑用堆维护。

而我们要同时维护最大值和最小值，可以用一个大根堆和一个小根堆。

AC代码

#include <bits/stdc++.h>
using namespace std;
const int N=20;
priority_queue<int,vector<int>,greater<int>>a;//大根堆
priority_queue<int,vector<int>,less<int>>b;//小根堆
int n;
int main(){
	cin>>n;
	for(int i=1;i<=n;i++){
		int t;
		cin>>t;
		a.push(t),b.push(t);//分别加入两个中
	}
	//分别进行两个堆的维护过程，具体过程很像果子合并
	while(a.size()>1){
		int tmp=a.top();
		a.pop();
		tmp*=a.top();
		a.pop();
		a.push(tmp+1);
	}
	while(b.size()>1){
		int tmp=b.top();
		b.pop();
		tmp*=b.top();
		b.pop();
		b.push(tmp+1);
	}
	cout<<a.top()-b.top();//输出题目要求维护的极差
}

【一本通提高篇贪心】 数列分段

题目链接

题目大意

给定的一个正整数数列 ，现要将其分成连续的若干段，并且每段和不超过$m$，问最少分成几段。

贪心策略

这题就是最简单的贪心了，枚举的过程中累加和，如果超过$m$就情况，然后段数$+1$，没有什么思维含量。

AC代码

#include <bits/stdc++.h>
using namespace std;
const int N=1e5+10;
int t,n,m,s=0,cnt=0;
int main(){
	cin>>n>>m;
	for(int i=1;i<=n;i++){
		cin>>t;
		if(s+t>m) s=0,cnt++;
		s+=t;
	}
	cout<<cnt+1;
}

【一本通提高篇贪心】 线段

题目链接

题目大意

在一个数轴上有$n$条线段，现要选取其中$k$条线段使得这$k$条线段两两没有重合部分,问最大的$k$为多少?

贪心策略

即不相交区间问题，和习题第一道几乎一模一样，这里直接上代码。

AC代码

#include <bits/stdc++.h>
using namespace std;
const int N=1e6+10;
struct node{
	int l,r;
}a[N];
bool cmp(node a,node b){
	return a.r<b.r;
}
int n,k=0;
int main(){
	scanf("%d",&n);
	for(int i=1;i<=n;i++) cin>>a[i].l>>a[i].r;
	sort(a+1,a+1+n,cmp);
	int t=0;
	for(int i=1;i<=n;i++){
		if(a[i].l>=t) t=a[i].r,k++;
	}
	cout<<k;
}

【一本通提高篇贪心】 家庭作业

题目链接

题目大意

有一些作业，每个作业有完成期限，每个作业完成会得到一定的学分，问最多能得到多少学分。

贪心策略

我们会发现这道题和智力大冲浪那题很像，但是那题做法的时间复杂度是$O(n^2)$，但这题的数据范围是$10^6$，所以需要优化。

一种优化方式是用并查集优化，并查集数组记录下最远能够追溯到的放置时间，如果用路径压缩优化的话，时间复杂度可以达到$O(\alpha n)$，其中$\alpha \le5$

AC代码

#include <bits/stdc++.h>
using namespace std;
const int N=1e6+10;
struct node{
	int t,x;
}a[N];
bool cmp(node a,node b){
	return a.x>b.x;
}
int n,m,tim[N],fa[N],ans=0;
int find(int x){
	//并查集查询
	if(x==fa[x]) return x;//路径压缩
	return fa[x]=find(fa[x]);
}
int main(){
	cin>>n;
	for(int i=1;i<=n;i++){
		cin>>a[i].t>>a[i].x;
		m=max(m,a[i].t);
	}
	for(int i=0;i<=m;i++) fa[i]=i;//初始化并查集
	sort(a+1,a+1+n,cmp);
	for(int i=1;i<=n;i++){
		int d=find(a[i].t);
		if(d) fa[d]=d-1,ans+=a[i].x;
	}
	cout<<ans;
}

【一本通提高篇贪心】 糖果传递

题目链接

题目大意

有$n$个小朋友坐成一圈，每人有$a_i$个糖果。每人只能给左右两人传递糖果。每人每次传递一个糖果代价为$1$。求使所有人获得均等糖果的最小代价。

贪心策略

本题需要一定的数学推导，比较繁琐，这里暂时略过，读者可以去找网上的题解。

AC代码

#include <bits/stdc++.h>
#define int long long
using namespace std;
const int N=1e6+10;
int n,a[N],s[N],sum=0;
signed main(){
	cin>>n;
	for(int i=1;i<=n;i++) cin>>a[i],sum+=a[i];
	for(int i=2;i<=n;i++){
		s[i]=s[i-1]+a[i]-sum/n;
	}
	sort(s+1,s+1+n);
	int mid=s[n/2+1],ans=0;
	for(int i=1;i<=n;i++) ans+=abs(s[i]-mid);
	cout<<ans;
}